HPCCprojects:Numerical modeling of 2D turbidity currents to investigate sediment wave generation
Numerical modeling of 2D turbidity currents to investigate sediment wave generation
Project description
Turbidity currents play an important role in the delivery of sediment to the deep seafloor. In some environments, repeated turbidity currents can result in sediment waves, large bedforms that appear similar to dunes but with wavelengths of up to several km. The waves are the result of greater deposition on the upstream/upslope side relative to the downstream side. This creates a distinctive upslope migration of the waveforms over successive currents that can be observed in profile. Field data for turbidity currents and their interaction with complex topographies such as sediment wave fields are very difficult to obtain. Thus, along with laboratory experiments, numerical simulation is an important means for investigating this phenomenon.
Previous modeling efforts have focused on using the depth-averaged formulation of the Navier-Stokes equations and small sets of input parameter combinations. For this investigation, we are using the 2D form of the Navier-Stokes equations in order to capture potentially important depth-wise flow structures. Additionally, we are applying a larger set of input parameter combinations in order to determine how the varying parameters affect the generation of sediment waves and the resulting morphology of any waves that may form. To do this we are using the 2D version of TURBINS. This code was developed by Mohamad M. Nasr Azadani to be capable of modeling gravity and turbidity currents interacting with complex topographies, including erosion.
We use a lock-exchange configuration in which a region of uniform concentration sediment-laden fluid initially sits atop a ramp. The parameters we have elected to vary for this study are settling velocity, Reynolds number, slope of the ramp, and height of the initial sediment-laden region of fluid relative to the lock. For each parameter combination, we simulate several successive flows, updating the bottom geometry between each flow. Thanks to the use of the immersed boundary method in the TURBINS code, even sub-grid changes to the bottom interface naturally affect the results of subsequent flows. In order to ensure that the mass input is the same for each simulation, the lock is cleared of deposition between runs. Over several flows, upstream-migrating waveforms can develop on the initially flat slope.
Objectives
- Use numerical simulations to predict the generation of sediment waves due to repeated turbidity currents
- Understand the influence of flow parameters (Reynolds number, etc.) on the resulting sediment wave morphology
Time-line
- Start: November 2011
- End: November 2013
Models in use
TURBINS, which has the following features:
- Navier-Stokes equations: to describe the fluid motion
- Transport equation(s): to describe the particle motion
- Immersed boundary method: to enforce accurate boundary conditions at the solid surface
- Multigrid solver is implemented via PETSc and HYPRE packages to solve for the descretized pressure Poisson equation
Results
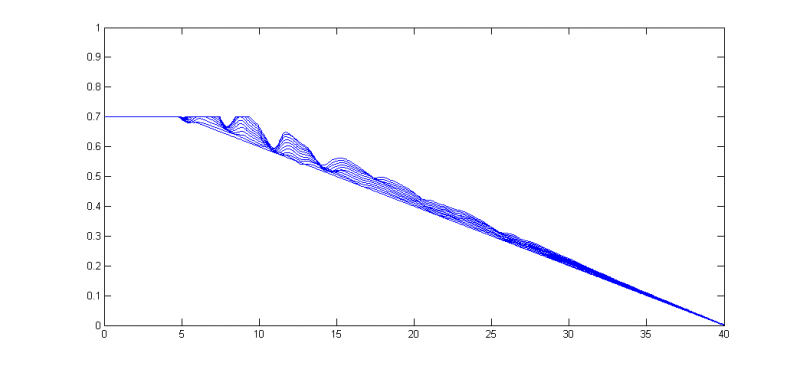
Deposit profiles for 10 repeated turbidity currents on an initially flat slope. Sediment-laden fluid filled a lock at the top of the slope from x=0 to x=5, and y=0.7 to y=2.7 (top of the y-domain).
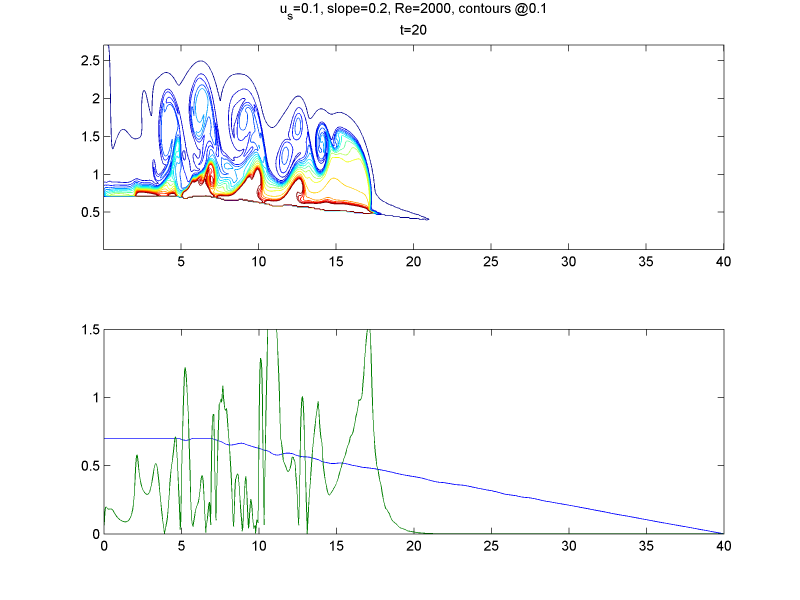
Snap-shot of flow over fourth deposit from first figure. (Top) Concentration contours at 0.1 interval (normalized to initial concentration within lock). (Bottom) Initial deposit (blue line) and shear stress at the bottom boundary, multiplied by 100 (green line).
For the above figures, non-dimensional particle settling velocity was set to 0.01, Reynolds number was 2000, and Schmidt number was 1.0. Half the lock height was used as the characteristic length scale to non-dimensionalize the Navier-Stokes equations.
Users
Funding
This work is funded by NSF award 1061300.
Publications and presentations
- G. Hoffmann, M.M. Nasr-Azadani, and E. Meiburg, Simulation of Sediment Wave Generation and Maintenance, AGU Fall Meeting, Dec. 2012
- G. Hoffmann, M.M. Nasr-Azadani, and E. Meiburg, Simulation of Sediment Wave Generation and Maintenance, 65th Annual APS Division of Fluid Dynamics Conference, Nov. 2012
Links
This would be the place to provide links that are related to your project.
Choose one of the two categories mentioned below, that your project suites the best